PIER M | PIER Journals

2013-07-10

PIER M

Vol. 31, 279-293, 2013

download: 208

A 3-D Unconditionally Stable Laguerre-Rpim Meshless Method for Time-Domain Electromagnetic Computations

Feijiao Liu , Donglin Su and Yilong Zhang

In this paper, a 3-D unconditionally stable meshless method is introduced to simulate time-domain electromagnetic problems. It combines the conventional radial point interpolation method (RPIM) and weighted decaying Laguerre polynomials together to discrete Maxwell's differential equations. The new method called Laguerre-RPIM retains the advantages of both the node-based meshless method and the unconditionally stable scheme of weighted Laguerre polynomials. The accuracy and efficiency of the proposed method are verified through two numeral examples. It can be seen from the computational results that the proposed method has a high accuracy and still remains stable when time step is 10 times of the Courant stability condition. Computational cost can be saved by more than 70% compared with the conventional RPIM method.

A 3-D UNCONDITIONALLY STABLE LAGUERRE-RPIM MESHLESS METHOD FOR TIME-DOMAIN ELECTROMAGNETIC COMPUTATIONS

2013-07-09

PIER M

Vol. 31, 263-278, 2013

doi:10.2528/PIERM13052019

download: 126

Simulation of SAR Under Ultra-Wide Band Electromagnetic Pulse in Human Tissue

Teng Jiao , Xiao Yu , Hao Lv , Yang Zhang , Hui Jun Xue , Yan Wang and Jianqi Wang

In this study, a three-dimensional (3-D) structural model of an adult male body, including 12 kinds of tissues and organs, was analyzed using a 3-D model design application (i.e., 3ds Max). The standard model of Asians was used as reference. The electrical parameters of brain tissues at different electromagnetic frequencies were introduced to obtain the electromagnetic model. Computational electromagnetic software based on the finite-difference time-domain was used to calculate the model absorption of electromagnetic waves under ultra-wide band electromagnetic irradiation. The specific absorption rates (SARs) of the ensemble average and the model human tissue were also obtained. This study aims to provide a parameter for the development of electromagnetic radiation protection standards, and to discuss related research.

SIMULATION OF SAR UNDER ULTRA-WIDE BAND ELECTROMAGNETIC PULSE IN HUMAN TISSUE

2013-07-08

PIER M

Vol. 31, 247-262, 2013

doi:10.2528/PIERM13052008

download: 225

Analysis and Modeling of Power MOSFET Radiation

Sofiene Saidi and Jaleleddine Ben Hadj Slama

This paper deals with the modeling the radiation of the power electronics component: the MOSFET. First, the magnetic near field measurements are made to characterize the radiation of the component. The MOSFET under test is referenced by IRF640 used in DC-DC converter. Second, we have applied the electromagnetic inverse method based on the measured field at 20 MHz to create a model of radiation sources of the MOSFET. The obtained results show a good agreement between the magnetic near field cartography obtained by the developed model and those measured. Finally, the developed model was used to predict the magnetic field in another distance and it wasvalidated with measured cartography.

ANALYSIS AND MODELING OF POWER MOSFET RADIATION

2013-07-08

PIER M

Vol. 31, 231-246, 2013

doi:10.2528/PIERM13050907

download: 159

Realization of Linear-to-Circular Polarization Conversion by a Single Bifilar Particle

Alexei Balmakou , Igor V. Semchenko and Masaaki Nagatsu

In this paper, we provide a new theoretical model describing mechanism of electromagnetic radiation (and scattering) by passive single- and double-stranded (bifilar) helices. The proposed model is derived from basic physical principles till the end formulas which were computer processed for predicting a polarization type of the wave scattered by a helix. Comparison of the two types of helical oscillators revealed radical differences in their scattering performance (intensity and polarization). Optimal parameters of the bifilar helix for transformation of the polarization state from linear to circular were found for a non-axial direction of the incident and scattered field. Key features of the proposed model were confirmed by computer simulations.

REALIZATION OF LINEAR-TO-CIRCULAR POLARIZATION CONVERSION BY A SINGLE BIFILAR PARTICLE

2013-06-30

PIER M

Vol. 31, 215-229, 2013

doi:10.2528/PIERM13041210

download: 137

Combined Method for Simulating Electron Spectrum of δ-Doped Quantum Wells in n -Si with Many-Body Corrections

Christian Castrejon-Martinez , Volodymyr V. Grimalsky , Luis Manuel Gaggero-Sager and Svetlana V. Koshevaya

The combined method to investigate the electron spectrum of single n-type d-doped quantum wells in silicon is proposed. It is based on computing the electron potential energy by means of the Thomas-Fermi method at finite temperatures; then the obtained electron potential energy is applied to the iteration procedure with solving the Schrodinger equations for the electron spectrum and the Poisson one for the potential energy. The many-body corrections to the electron spectrum in the quantum well also have been investigated. The combined method demonstrates a rapid convergence. It is shown that that the simple TF method gives a good approximation for the electron potential energy and for the total electron concentration within the well.

COMBINED METHOD FOR SIMULATING ELECTRON SPECTRUM OF δ-DOPED QUANTUM WELLS IN N-SI WITH MANY-BODY CORRECTIONS

2013-06-29

PIER M

Vol. 31, 199-214, 2013

doi:10.2528/PIERM13051311

download: 1289

Conductor Fusing and Gapping for Bond Wires

Kenneth Chien-Ying Chen , Larry Kevin Warne , Yau Tang Lin , Robert L. Kinzel , Johnathon D. Huff , Michael B. McLean , Mark W. Jenkins and Brian M. Rutherford

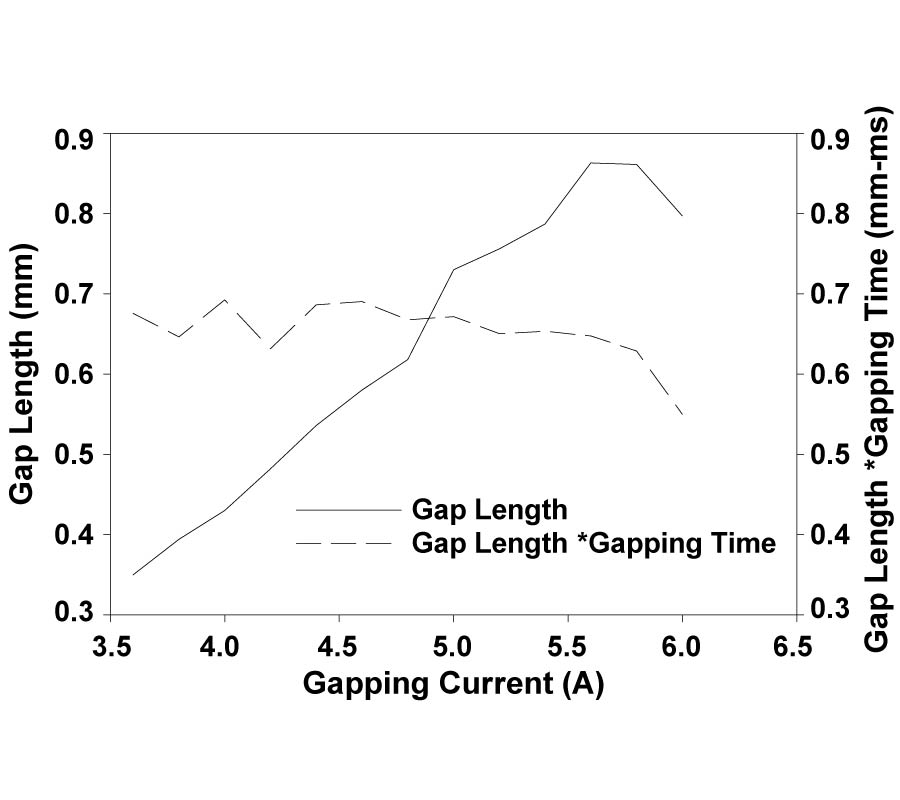
In this paper, fusing of a metallic conductor is studied by judiciously using the solution of the one-dimensional heat equation, resulting in an approximate method for determining the threshold fusing current. The action is defined as an integration of the square of the wire current over time. The burst action (the action required to completely vaporize the material) for an exploding wire is then used to estimate the typical wire gapping action (involving wire fusing), from which gapping time can be estimated for a gapping current greater than a factor of two over the fusing current. The test data are used to determine the gapped length as a function of gapping current and to show, for a limited range, that the gapped length is inversely proportional to gapping time. The gapping length can be used as a signature of the fault current level in microelectronic circuits.

CONDUCTOR FUSING AND GAPPING FOR BOND WIRES

2013-06-27

PIER M

Vol. 31, 189-198, 2013

doi:10.2528/PIERM13050510

download: 186

Simplified Modeling and Measurement Procedures for Electromagnetic Launchers Performances Evaluation

Hocine Menana

In this work, Simplified modeling and measurement procedures for capacitive driven electromagnetic launchers using magnetic armatures are presented. The modeling strategy is based on a successive solving of the circuit equation coupled to a 2D finite element (FEM) magnetostatic computation and the mechanical equation of the armature motion. This leads to a considerable time and memory space saving compared to a time domain magnetodynamic problem computation. The armature velocity is determined through the analysis of the time variation of the induced voltage, due to the armature remanent magnetization, in an auxiliary coil placed at the launcher extremity. The modelling and measurement strategies are implemented and tested on a laboratory developed coil-gun prototype. Modelling and measurement results are provided.

SIMPLIFIED MODELING AND MEASUREMENT PROCEDURES FOR ELECTROMAGNETIC LAUNCHERS PERFORMANCES EVALUATION

2013-06-26

PIER M

Vol. 31, 171-188, 2013

doi:10.2528/PIERM13050113

download: 155

The RFI Suppression Method Based on Stft Applied to SAR

Tengfei Zhao , Yongsheng Zhang , Lin Yang , Zhen Dong and Diannong Liang

Improved radio frequency interference suppression method based on short time Fourier transform applied to synthetic aperture radar is proposed in this paper. The radio frequency interference, including narrow-band interference and wide-band interference, are analyzed in time frequency domain. The interference is identified at instantaneous frequency spectrum by a novel threshold criterion in time frequency domain, and then an adaptive gain coefficient is determined for instantaneous frequency spectrum at every certain time. The gain coefficient can keep the useful signal correctly during interference suppression. In the end, the performance of the proposed method is demonstrated by the experiment based on the real synthetic aperture radar data adding the interference.

THE RFI SUPPRESSION METHOD BASED ON STFT APPLIED TO SAR

2013-06-14

PIER M

Vol. 31, 159-169, 2013

doi:10.2528/PIERM13042312

download: 154

Proposing a Wavelet Based Meshless Method for Simulation of Conducting Materials

Arman Afsari and Masoud Movahhedi

This work focuses on the development of multiscale meshless technique in area of scattered fields from paramagnetic scatterers. The radial point interpolation method (RPIM), as the most common meshless technique, is employed for above purpose. Due to high frequency analysis, some special considerations must be applied, particularly in subdomains near the incident face. So, to ensure the accuracy, a multiscale meshless technique in wavelet frames sounds necessary. Simulating the scatterers using above method, specifically an elliptic paramagnetic scatterer, shows some efficient aspects such as less computational time and more precision compared with some other numerical methods.

PROPOSING A WAVELET BASED MESHLESS METHOD FOR SIMULATION OF CONDUCTING MATERIALS

2013-06-14

PIER M

Vol. 31, 143-158, 2013

doi:10.2528/PIERM13041301

download: 166

Design Optimization of a Novel Magnetic Switchable Device Based on Halbach Array

Yong Chen , Changming Wang and Jiandong Bao

The paper deals with the design and optimization of a novel magnetic switchable device based on Halbach array. The magnetic field in air gap is adjustable by rotating the center axis of adhesion mechanism so that the magnetic adhesion force is variable, and it is convenient for device to adsorb on and detach from the ferromagnetic workpiece or surface. The magnetic field model is established by Fourier series method, and the optimal dimensions of configuration are obtained by finite element parameter approximation method for best performing design. The magnetic force of novel optimal device is measured, and a good agreement between simulation and measurement is found. The results are compared to the traditional mechanism, and it is shown that the utilization ratio of magnets of novel optimal mechanism is 2.2 times larger than the H-type one with the same usage of magnets, while its consumption of soft iron is only 12.7% of the H-type one.

DESIGN OPTIMIZATION OF A NOVEL MAGNETIC SWITCHABLE DEVICE BASED ON HALBACH ARRAY

2013-06-11

PIER M

Vol. 31, 129-141, 2013

doi:10.2528/PIERM13041607

download: 259

A Methodology for the Design of Microwave Systems and Circuits Using an Evolutionary Algorithm

Massimo Donelli , Md. Rukanuzzaman and Carlos E. Saavedra

This work presents a methodology for the development of microwave systems and circuits. Starting from the system decomposition, the proposed method is aimed at estimates the requirements of each component of the system taking into account the effects on the whole system and the interactions with the others microwave components. The obtained requirements are then used to design or optimize each device with standard design methodologies or CAD tools. The problem is recast as an optimization one by defining a suitable cost function able to take into account the interactions between all the components of the system. The cost function is then minimized with an evolutionary optimization technique, namely the particle swarm optimizer. The obtained preliminary results, concerning the design of a broad-band bidirectional amplifier, demonstrate the potentialities of the proposed approach.

A METHODOLOGY FOR THE DESIGN OF MICROWAVE SYSTEMS AND CIRCUITS USING AN EVOLUTIONARY ALGORITHM

2013-06-03

PIER M

Vol. 31, 117-127, 2013

doi:10.2528/PIERM13041902

download: 156

Improved Immunity Measurement of a Microcontroller to Conducted Continuous Wave Interference

Fayu Wan , Jun-Xiang Ge , Yong Zhou and Bing Yu

This paper discusses an improved in-situ immunity measurement test bench of a microcontroller -PIC18F458 to conducted continuous wave interference (CWI). The updated measurement algorithm gives more accurate measurement result. Compared with normal failure criterion, the DC shift failure criterion is adopted because it gives better description of the immunity behavior of the microcontroller. Finally, the susceptibility results are explained in detail.

IMPROVED IMMUNITY MEASUREMENT OF A MICROCONTROLLER TO CONDUCTED CONTINUOUS WAVE INTERFERENCE

2013-05-30

PIER M

Vol. 31, 103-116, 2013

doi:10.2528/PIERM13022201

download: 178

Design of a Low Sidelobe 4D Planar Array Including Mutual Coupling

Quanjiang Zhu , Shiwen Yang , Ruilin Yao and Zai-Ping Nie

An efficient approach is presented for the design of a low sidelobe four-dimensional (4D) planar antenna array, taking into account mutual coupling and platform effect. The approach is based on the combination of the active element patterns and the differential evolution (DE) algorithm. Different from linear and circular arrays, the mutual coupling compensation in a planar array is more complicated since it requires numerous data of the active element patterns in different azimuth planes. In order to solve this problem, a useful interface program is developed to get these data from commercial software HFSS automatically. Also different from conventional low sidelobe arrays with tapered amplitude excitations, the low sidelobe in the 4D array is realized using time-modulation technique under uniform static amplitude and phase conditions. The DE algorithm is used to optimize the time sequences which are equivalent to the complex excitations in conventional arrays. Both computed results and simulated results in HFSS show that a -30 dB sidelobe pattern can be synthesized in a 76-element planar array with an octagonal ground plane and a radome, thus verifying the proposed approach.

DESIGN OF A LOW SIDELOBE 4D PLANAR ARRAY INCLUDING MUTUAL COUPLING

2013-05-30

PIER M

Vol. 31, 85-101, 2013

doi:10.2528/PIERM12110303

download: 136

Aircraft-Lightning Electrodynamics Using the Transmission Line Model Part I: Review of the Transmission Line Model

Sanmugasundaram Thirukumaran , Paul Ratnamahilan Polycarp Hoole , Ramiah Harikrishnan , Jeevan Kanesan , Kandasamy Pirapaharan and Samuel Ratnajeevan Herbert Hoole

This work presents a self-consistent and self-contained model to study and analyze aircraft-lightning electrodynamics. In this paper we review the well developed and reported transmission line model of the cloud-to-ground (CG) lightning return stroke. Subsequently, the incorporation of a circuit model of the aircraft into the return stroke model is considered. The direct hit characteristics of aircraft body lightning currents for both CG and GC (ground-to-cloud) are important when designing protection, shielding and filtering systems for airborne electronic and electrical systems within the aircraft system. Moreover, the model will allow design of aircraft structure and geometry to minimize energy dissipation into the aircraft structure and systems. Basic electromagnetic theory is used to show the validity of considering the return stroke as a transverse magnetic wave along a transmission line. A distributed transmission line model for the aircraft and the return stroke channel of the lightning is used to simulate the return strokes of CG and GC flashes. The effects of the aircraft geometry with sharp edges are included in the computation of aircraft capacitance values, both distributed as well as lumped values. The paper compares electric currents, channel voltages, the rate of change of current and the frequency spectrum along the lightning channel of the return strokes for CG and GC flashes with aircraft attached to the channel.

AIRCRAFT-LIGHTNING ELECTRODYNAMICS USING THE TRANSMISSION LINE MODEL PART I: REVIEW OF THE TRANSMISSION LINE MODEL

2013-05-30

PIER M

Vol. 31, 71-83, 2013

doi:10.2528/PIERM13040804

download: 132

A Hybrid Method of Scattering from a Dielectric Target Above a Rough Surface: TE Case

Lin Li , Tian Lin Dong and Qingxia Li

A hybrid method, combining analytic Kirchhoff approximation (KA) and numerical method of moments (MoMs), is developed to solve the two-dimensional (2D) scattering problem of a dielectric target with arbitrary cross section above a moderate perfect electric conductor (PEC) rough surface under TE-polarized tapered wave incidence. The induced current on the rough surface is analytically expressed using the KA method, which depends on the tapered incident wave and the field illuminating by current distribution on the target, leaving only unknown induced current on the target. So the electric field integral equations of the induced currents on the target only can be derived; it allows a substantial reduction of computation time and memory requirement. Furthermore, for different secondary scattering of the underlying rough surface, different truncations of the rough surface are taken to speed up computation of the scattering contribution from the rough surface to the target. Making use of Monte Carlo realization, bistatic scattering from a cylindrical target above a PEC rough surface is well simulated to test validity and efficiency of the proposed method. Numerical results from the hybrid method have good agreements with those from the conventional method of moments. However, the computational time and the memory requirements have been greatly reduced.

A HYBRID METHOD OF SCATTERING FROM A DIELECTRIC TARGET ABOVE A ROUGH SURFACE: TE CASE

2013-05-29

PIER M

Vol. 31, 59-69, 2013

doi:10.2528/PIERM13042409

download: 146

Dual-Band Terahertz Chiral Metamaterial with Giant Optical Activity and Negative Refractive Index Based on Cross-Wire Strucure

Fang Fang and Yongzhi Cheng

In this paper, a dual-band chiral metamaterial (CMM) based on cross-wire structure is proposed and studied numerically. It exhibits dual-band giant optical activity and negative refractive index in terahertz region. The surface current distributions are calculated to explain original physics. The further numerical results show that the effective frequency bands of the CMMs can be independent adjusted easily by changing the structure geometrical parameter. The designed dual-band terahertz CMMs offer ﬂexibility in the investigation of novel terahertz device application.

DUAL-BAND TERAHERTZ CHIRAL METAMATERIAL WITH GIANT OPTICAL ACTIVITY AND NEGATIVE REFRACTIVE INDEX BASED ON CROSS-WIRE STRUCURE

2013-05-26

PIER M

Vol. 31, 45-58, 2013

doi:10.2528/PIERM13032801

download: 170

Time Reversal for Soft Faults Diagnosis in Wire Networks

Lola El Sahmarany , Laure Berry , Nicolas Ravot , Fabrice Auzanneau and Pierre Bonnet

Time-reversal (TR) invariance of the wave equation in lossless transmission line (TL) is here introduced as an improvement for fault-detection techniques in wire networks. This new approach is applied to reflectometry in wire diagnosis. To test the efficiency of this method, the reverse time algorithm simulated with FDTD (Finite Difference Time Domain) is developed in a one dimension space. It uses a new signal processing and an adapted signal to the wire under test for diagnosing the fault in the wire. In addition the interest of the convolution product between the incident signal and the output signal from this reverse time method will be also shown and applied in this paper. Through numerical simulations and experimental results measured on coaxial cable, the benefits of this method have been illustrated.

TIME REVERSAL FOR SOFT FAULTS DIAGNOSIS IN WIRE NETWORKS

2013-05-23

PIER M

Vol. 31, 29-43, 2013

doi:10.2528/PIERM13042405

download: 186

On the Success of Electromagnetic Analytical Approaches to Full Time-Domain Formulation of Skin Effect Phenomena

Jose Antonio Marinho Brandao Faria and Malcolm Stuart Raven

Maxwell equations can be used to formulate an analytical full time-domain theory of skin effect phenomena in circular cylindrical conductors without any detour into the frequency domain. The paper shows how this can be done and concomitantly provides the means to determine the time-varying per unit length voltage drop along the conductor from a given time-varying conductor current. The developed relationship between voltage and current is not very complicated and led the authors to examine the reasons why it has never been utilized in transient analysis, nor given special emphasis in the literature. Those reasons are thoroughly examined and the conclusion is that the conditions required for the application of a purely time-domain skin effect theory are very restrictive.

2013-05-19

PIER M

Vol. 31, 13-27, 2013

doi:10.2528/PIERM13041601

download: 199

A Loop-Type End-Launcher for Carbon Fiber Reinforced Polymer Waveguides

Alexe Bojovschi , Derek Gray and Kamran Ghorbani

The analysis of an end-launcher type transition from coaxial to WR90 waveguides is presented. This transition is tuned to have the highest performance at the radar frequency of 9.375 GHz. The characteristics of the transducer are investigated comparatively in 30 cm aluminum and carbon fiber reinforced polymer waveguides. The advantage of the proposed feed is that it does not require grounding to the broad wall of the waveguide compared to the traditional end-launcher loop feeds. This departure from the current loop feeds makes the proposed feed suitable for carbon fiber reinforced polymer waveguides where a disruption in the broad wall would be undesirable.

A LOOP-TYPE END-LAUNCHER FOR CARBON FIBER REINFORCED POLYMER WAVEGUIDES

2013-05-15

PIER M

Vol. 31, 1-11, 2013

doi:10.2528/PIERM13031909

download: 151

Terrain Height Estimation Using a Stereo-SAR Technique Aided by a Reference Point

Kung-Yu Lu and Jean-Fu Kiang

A stereo-synthetic aperture radar (stereo-SAR) technique is proposed to estimate the terrain height of a target area. A reference point with known altitude is located within the target area to calibrate the height estimation. The estimated height error can be reduced to one meter. This method requires the processing techniques of conventional SAR, while achieving a fairly fine resolution in height estimation for practical applications.